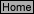R-3
for which n = 2 and E° = -0.76 V. The electrode potential represents the tendency for Zn
2+
to be
reduced to Zn(s) if all concentrations in the half-cell are 1.00 M. For this example, Q is 1/[Zn
2+
].
(Note that the concentration of Zn(s) does not appear in Q because it is a solid.) The Nernst equation
for this half-cell is:
E
half-cell
= -0.76 V -
0.0592
2
log
1
2
[
]
Zn
(6)
II. Calculations Using the Nernst Equation
A. Spontaneous Cell Reactions
To review the information from Experiment Q, any two half-cells can be combined to form an
electrochemical cell. Electrons given off in one half-cell must equal those gained by the other. For the
cell reaction to occur spontaneously, E
cell
must be positive. When half-cell reactions are written as
reduction processes, and are ordered in a displacement series, so that the better reducing agent is at the
top (electrode potential is more negative), an equation for a spontaneous reaction will always result by
taking a half-cell reaction and adding to it any oxidation half-cell reaction above it. An oxidation
reaction is simply the reverse of a reduction reaction. If the direction of the reaction is reversed then the
sign on the half-cell potential must also be reversed. In a voltaic cell the half-cell with the more positive
reduction potential will be the reduction (cathode) half-cell and the other half-cell will be the oxidation
(anode) half-cell. The cell potential will be the difference of the two standard reduction potentials:
E
cell
= E
red, cathode
- E
red, anode
(7)
B. Calculation of the Cell Potential at Nonstandard Conditions
There are two methods that can be used to calculate the (overall) cell potential. In the first method, a
balanced chemical equation for the overall cell reaction is written and then the Nernst equation is written
for it as:
E
cell
= E
cell
-
0.0592
n
log Q
cell
(8)