N-6
the lid, and hold the thermometer in place with a rubber band to form the top assembly piece. By
moving this rubber band, the position of the thermometer will be adjusted so that the bulb is about 1 cm
above the bottom of the calorimeter when the top assembly is put into the cups.
The heat evolved during the reaction will be absorbed by the water of the aqueous solution, the solutes
dissolved in the aqueous solution, the thermometer, the polystyrene cups, the polystyrene cover, and
(since no insulation is perfect) the air around the calorimeter. By far, the greatest portion of the heat will
be absorbed by the water. This is because the specific heat capacity of water (4.184 J/g
C) is much
greater than that of the other components. Hence, in this experiment you will assume that all of the heat
released (absorbed) by the reaction is absorbed (released) by the water.
This means that for an exothermic reaction, as soon as the temperature of the reaction solution begins to
rise, heat is lost to parts of the calorimeter and to the atmosphere. As a result, the maximum temperature
change measured in your experiments will be less than the temperature change you would have
measured, if there had been no loss of heat from the calorimeter. After the maximum temperature is
reached, you will observe a slow drop in temperature with time. This drop in temperature corresponds
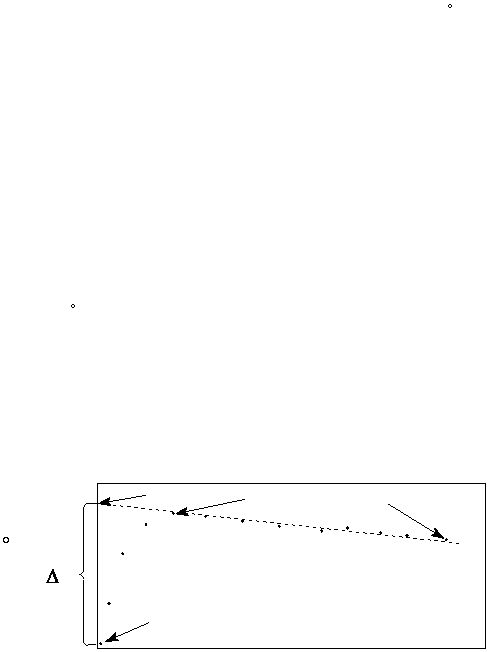
to the rate at which heat is being lost by the calorimeter to the surroundings. If you plot a graph of the
measured temperature, t (in
C), against time and extrapolate back to time = 0 (note: time = 0 is the
time at which the reagents were mixed), you will get the corrected final temperature, which correlates to
the extrapolated temperature (t
e
) (see Fig. 2).
The value of t
e
is used to calculate the corrected
?t, which is the change that would have occurred, if the calorimeter were perfectly insulated
(i.e. no heat loss to the surroundings). (?t = t
e
- t
i
)
t
f
_
_
_
_
| | | | | | | | | | | | | |
Time
Temperature
C
t
e
t
i
t
max
t
Figure 2